Given:

Present Value = Rs. 11,979

Interest rate = 10% per annum

Time = 3 years

Concept:

Compound Interest formula, 

Amount. =  P(1 + r/100)n 

Solution:

Let the borrowed money (Principal) be P.

We have the formula for compound interest, P(1 + r/100)ⁿ = Amount.

Here, P(1 + 10/100)³ = 11,979

Solving for P, we get P = 11,979/(1.1)³ = Rs. 9,000.

Hence, Rahul borrowed Rs. 9,000.

Given: 

10 women can do a work in 6 days.

6 men can do the same work in 5 days.

Formula Used:

Work Done = time × efficiency

Calculation:

⇒ 10W × 6 = 6M × 5

⇒ 60W = 30M

⇒ W/M = 1/2

Total Work = 60

Time = 60/(10 × 1 + 6 × 2)

⇒ 60/22 = 30/11

Given:

BD = 3 cm

DA = 4 cm

Concept used:

Concept of similarity

Calculation:

Here ΔABC ∼ ΔBDE [∵ DE || AC and ∠B is common for two triangles]

So,

ΔABC/ΔBDE = 7²/3²

⇒ ΔABC/ΔBDE = 49/9

ACED = ΔABC - ΔBDE

⇒ 49 - 9 = 40

∴ Ratio is 9 : 40

∴ Then Area of ΔBDE : Area of trapezium ACED is 9 : 40

Given:

Concept used:

Calculation:

Given:

19¹⁹ + 20

Concept used:

A ≡ B (Mod C) means A leaves a remainder of B when divided by C.

Calculation:

We know,

19 ≡ 1 (Mod 18)

⇒ 19¹⁹ ≡ 1¹⁹ (Mod 18)

⇒ 19¹⁹ ≡ 1 (Mod 18)

When 20 is divided by 18, the remainder = 2

(19¹⁹ + 20) ≡ (1 + 2) (Mod 18)

⇒ (19¹⁹ + 20) ≡ 3 (Mod 18)

∴ The remainder when 19¹⁹ + 20 is divided by 18, is 3.

∠ABC = ∠BAC = 50° [AC = BC]

In ΔABC

∠ABC + ∠BAC + ∠ACB = 180°

⇒ 50° + 50° + ∠ACB = 180°

⇒ ∠ACB = 180° - 100°

⇒ ∠ACB = 80°

Now, ∠ACB + ∠ACD = 180°

⇒ ∠ACD = 180° - 80° = 100°

Now, ∠CAD = ∠CDA [AC = BC = CD]

In ΔACD

∠CAD + ∠CDA + ∠ACD = 180°

⇒ 2∠CAD + 100° = 180°

⇒ 2∠CAD = 180 - 100 = 80

⇒ ∠CAD = 80°/2 = 40°

Now, ∠BAD = ∠BAC + ∠CAD

∴ ∠BAD = 50° + 40° = 90°

Given:

Pipes A and B can fill a tank in 36 minutes and 45 minutes

Concept used:

Total work = Time taken by the pipes × efficiency of the pipes

Calculation:

Let the efficiency of the leakage be (- x) units/minute

LCM of 36 and 45 = 180

So, Let the total work be 180 units

Now, efficiency of pipe A = 180/36 = 5 units/minute

And, efficiency of pipe B = 180/45 = 4 units/minute

According to the question,

All three pipes were opened for the first 20 minutes, and then the leakage pipe was sealed and the tank was full in 15 minutes.

i.e., (9 - x) × 20 + 15 × 9 = 180

⇒ 180 - 20x + 135 = 180

⇒ - 20x = - 135

⇒ x = 135/20 or 27/4

Now, time taken by the leakage pipe to empty the tank = Total work\Efficiency

⇒ 180/(27/4) ⇒ 180 × (4/27)

⇒ 80/3

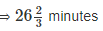
∴ The leak alone can empty the full tank in 

Calculation:

Students who obtained less than 50% marks approximately = 10

Students who obtained more than 90% marks and above = 25

Difference = 25 - 10 = 15

Given:

PS = 12 cm

NR = 9 cm

QM = NR and NR = SN

Formula Used:

Area of Trapezium = (1/2) × (Sum of Parallel Sides) × Height

Calculation:

According to the question,

QM = NR and NR = SN

⇒ QM = NR = SN = 9 cm

Again according to the question,

PM || SN

⇒ PS = MN = 12 cm

In trapezium PQRS,

QR = QM + MN + NR

⇒ QR = 9 + 12 + 9 = 30 cm

Height = SN = 9 cm

Area of Trapezium = (1/2) × (PS + QR) × SN

⇒ Area of Trapezium = (1/2) × (12 + 30) × 9

⇒ Area of Trapezium = (1/2) × (12 + 30) × 9

⇒ Area of Trapezium = 189 cm²