Now using componendo-dividendo,

Now, at x = 1

Given:

f(x) = (x² - 4)(x - 1)² and g(x) = x²

Calculation:

Given:

f (x) = 1 + x + x² + x³ +…+ x¹⁰⁰, g (x) = 6x

Calculation:

f (x) = 1 + x + x² + x³ +…+ x¹⁰⁰

Now,

Given:

f (x) = 1 + x + x² + x³ +…+ x¹⁰⁰, g (x) = 6x

Formula Used:

The sum of a series 1, 2, 3 ......n is given by

Calculation:

f (x) = 1 + x + x² + x³ +…+ x¹⁰⁰

⇒ f'(x) = 0 + 1 + 2x + 3x² +…+ 100x⁹⁹

⇒ f'(x) = 1 + 2x + 3x² +…+ 100x⁹⁹

Now,

Concept:

Real Functions:

If f(x) = a⁰x⁰ + a¹x¹ + a²x² + ... anxn, where a⁰, a¹, ... an are constants, then, f(y) = a⁰y⁰ + a¹y¹ + a²y² + ... anyn.

Calculation:

Concept:

If f: A → B and g: B → C are functions then g o f (x) = g( f (x)) is a function from A to C.

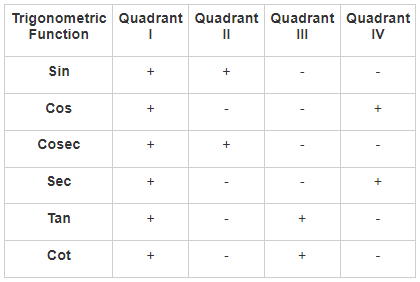
Sign of trigonometric functions in different quadrants is as shown below:

Calculation:

Given: f(x) = |x| and g(x) = cos x 

As we know that, if f: A → B and g: B → C are functions then g o f (x) = g( f (x)) is a function from A to C.

So, g o f(3π/4) = g (f (- 3π/4))

∵ f(x) = |x| so, f(- 3π/4) = 3π/4

⇒ g o f(3π/4) = g(3π/4)

∵ g(x) = cos x so, g(3π/4) = cos (3π/4) = cos (π - (π/4))

As we know that, cos (π - θ) = - cos θ

∴ g o f(3π/4) = - cos (π/4) = - √ 2/2------------(1)

Similarly, g o f(5π/6) =g (f (5π/6))

∵ f(x) = |x| so, f(5π/6) = 5π/6

⇒ g o f(5π/6) = g(5π/6)

∵ g(x) = cos x so, g(5π/6) = cos (5π/6) = cos (π - (π/6))

As we know that, cos (π - θ) = - cos θ

∴ g o f(5π/6) = - cos (π/6) = - √3/2---------------(2)

From equation (1) and (2), we get

⇒ g o f(- 3π/4) + g o f(5π/6) = (- √2/2) + (- √3/2)

Given:

A parabola passes through (1, 2) and satisfies the differential equation

Concept:

The general equation of a parabola where (h, k) denotes the vertex is

y = a (x - h)² + k, the directrix of the parabola is y = k - 1/4a

x = a (y - k)² + h, the directrix of the parabola is x = h - 1/4a

Calculation:

Parabola passes through (3, 2)

So, x = 3, y = 2

2 = c (3 - 2)²

c = 2

Now comparing the given equation of a parabola with the general form y = a (x - h)² + k, 

Here, (h, k) is (2, 0) and a = 2

The directrix of the parabola is y = k - 1/4a

Given:

A parabola passes through (1, 2) and satisfies the differential equation

Concept:

The general equation of a parabola where (h, k) denotes the vertex is

y = a (x - h)² + k, Vertex (h, k), Focus (h, k + (1/4a))

x = a (y - k)² + h, Vertex (h, k), Focus (h + (1/4a), k)

Calculation:

Parabola passes through (3, 2)

So, x = 3, y = 2

2 = c (3 - 2)²

c = 2

Now comparing the given equation of a parabola with the general form y = a (x - h)2 + k, 

Here, (h, k) is (2, 0), and a = 2

Vertex = (2, 0)​

Focus = (h, k + (1/4a)) = (2, 1/8)

∴ The correct answer is option (3).

Concept:

L-Hospital Rule: Let f(x) and g(x) be two functions

Suppose that we have one of the following cases,

Note: We have to differentiate both the numerator and denominator with respect to x unless and until