A particle executes simple harmonic motion with an amplitude of 4cm. At the mean position, velocity of the particle is 10cm∕ s. The distance of the particle from the mean position when its speed becomes 5cm∕ s is √α cm, where α = ___
[27-Jan-2024 Shift 1]
A ball suspended by a thread swings in a vertical plane so that its magnitude of acceleration in the extreme position and lowest position are equal. The angle (θ) of thread deflection in the extreme position will be :
[27-Jan-2024 Shift 2]
When the displacement of a simple harmonic oscillator is one third of its amplitude, the ratio of total energy to the kinetic energy is x/8, where x =____
[29-Jan-2024 Shift 1]
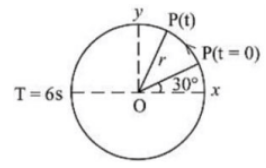
A simple harmonic oscillator has an amplitude A and time period 6π second. Assuming the oscillation starts from its mean position, the time required by it to travel from x = A to x = √3/2 A will be π/x s, where x = _____:
[29-Jan-2024 Shift 2]
A simple pendulum is placed at a place where its distance from the earth's surface is equal to the radius of the earth. If the length of the string is 4m, then the time period of small oscillations will be _____s. [. take g = π2 ms−2]
[30-Jan-2024 Shift 2]
A particle performs simple harmonic motion with amplitude A. Its speed is increased to three times at an instant when its displacement is 2A/3. The new amplitude of motion is nA/3. The value of n is___
[31-Jan-2024 Shift 1]
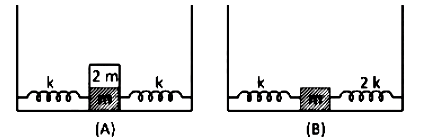
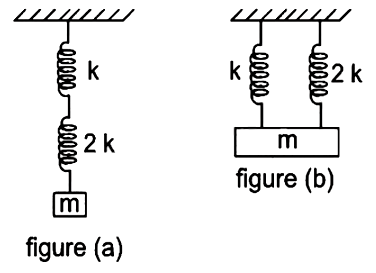
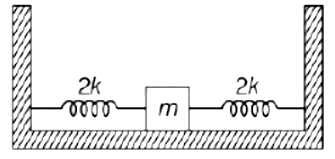
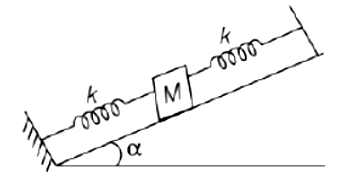
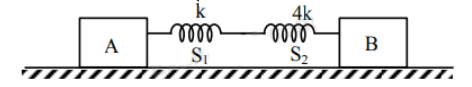
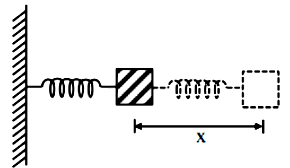
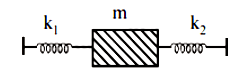
The time period of simple harmonic motion of mass M in the given figure is π  where the value of α is _____
where the value of α is _____
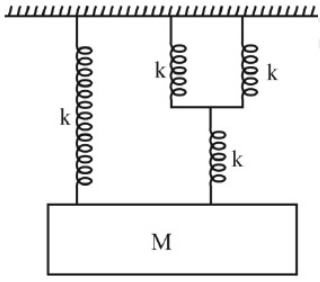
[31-Jan-2024 Shift 2]
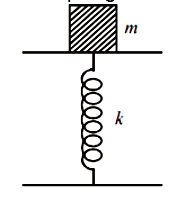
A mass m is suspended from a spring of negligible mass and the system oscillates with a frequency f1. The frequency of oscillations if a mass 9m is suspended from the same spring is f2. The value of f1/f2 is:
[1-Feb-2024 Shift 2]

 ×
×